Education

I was reading a book on ancient mathematics problems from Indian mathematicians. Here I wish to share one problem from Bhaskaracharya‘s famous creation Lilavati. Who was Bhaskaracharya? Bhaskara II, who is popularly known as Bhaskaracharya, was an Indian mathematician and astronomer from the 12th century. He’s especially known for the discovery of the fundamentals of…

Henry Poincaré was trying to save the Old classical theory of Physics by Suitable Adjustments & Modifications in it. When the experiments, like Michelson Morley Experiment, in search of the ether drift failed, it began to be increasingly realized that there was no such thing as an absolute or privileged frame of reference and that…

The Collatz Conjecture is one of the Unsolved problems in mathematics, especially in Number Theory. The Collatz Conjecture is also termed as 3n+1 conjecture, Ulam Conjecture, Kakutani’s Problem, Thwaites Conjecture, Hasse’s Algorithm, and Syracuse Problem. Collatz Conjecture Statement If you keep repeating this procedure, you shall reach the number 1 at last. Illustrations » Starting…

Topic: Beta & Gamma functions The Gamma function and Beta functions belong to the category of special transcendental functions and are defined in terms of improper definite integrals. Definitions of Beta and Gamma functions are given below. But before that, let’s quote the statement of Dirichlet’s theorem so that we can work around Liouville’s extension…

In this article, we will learn about the Lindemann Theory of Unimolecular Reactions, which is also known as the Lindemann-Hinshelwood mechanism. Understanding Lindemann Theory of Unimolecular Reactions It is easy to understand a bimolecular reaction on the basis of collision theory. When two molecules A and B collide, their relative kinetic energy exceeds the threshold…

In a busy marketplace, two women sell marbles differently: one sells three marbles for a Rupee, and the other sells two marbles for a Rupee. When they combine their unsold marbles and try to sell them together, they expect to make the same amount of money. But to their surprise, they end up one Rupee…

In 1904, the french Mathematician Henri Poincaré (en-US: Henri Poincare) posed an epoch-making question, which later came to be termed as Poincare Conjecture, in one of his papers, which asked: If a three-dimensional shape is simply connected, is it homeomorphic to the three-dimensional sphere? Henri Poincare – 1904 So what does it really mean? How…

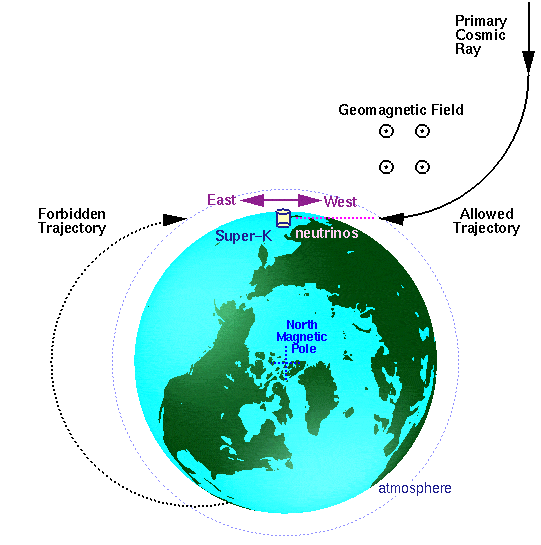
Wondering what Cosmic Radiation & Cosmic Ray Showers are and how these are formed? If you are, you are at the perfect place. Cosmic radiation and cosmic ray showers are some of the most intriguing and fascinating phenomena in the universe. While the term “radiation” may have negative connotations, cosmic radiation is a natural occurrence…

Lord Rayleigh made an attempt to explain the energy distribution in black body radiation, which was completed by Jeans in 1900. The results obtained by then are known as Rayleigh-Jeans’ Rules on Black Body Radiation. The law covering these rules is called Rayleigh Jean’s Law. The black body emits radiation of continuously variable wavelengths right…

Various physicists tried to explain the problem of energy distribution in black body radiation, and finally, German Physicist Max Planck successfully solved the problem. Before him, German Physicist Wilhelm Wein and British Physicist Lord Rayleigh & James Jean have tackled this problem and have given important laws. In fact, the work of their scientists paved…

Derivative of x squared As we know, the derivative of x squared, i.e., differentiation of $ x^2$ , with respect to $ x$, is $ 2x$. i.e., $ \dfrac{d}{dx} x^2 = 2x$ A Curious Case Suppose we write $ x^2$ as the sum of $ x$ ‘s written up $ x$ times. i.e., $ x^2…

Consider a sequence of functions as follows:- $ f_1 (x) = \sqrt {1+\sqrt {x} } $ $ f_2 (x) = \sqrt{1+ \sqrt {1+2 \sqrt {x} } } $ $ f_3 (x) = \sqrt {1+ \sqrt {1+2 \sqrt {1+3 \sqrt {x} } } } $ ……and so on to $ f_n (x) = \sqrt {1+\sqrt{1+2 \sqrt…